The Insanity of Compound Interest

Main points:

• We cannot expect our pension funds to keep growing -- therefore, they will be inadequate for our needs
• Compound interest cannot be part of a stable, sustainable society
• Long term investments such as power plants financed with long term debt will end badly

We take for granted the concept that our lifetime savings will grow and provide for our retirement and that there are interest payments on bank loans. Unfortunately, there is a fatal flaw.

Let's begin with Julias Caesar. Somehow he got ahold of a Canadian loonie ($1) and bought Canada savings bonds yielding 10%. Today he checks it out and finds, 2050 years later that it has grown to:

$2,761,071,942,588,640,000,000,000,000,000,000,000,000,000,000,000,000,000,000,000,000,000,000,000,000,000,000,000.00

Aside from the fact, that the coins would now weigh more than the known universe, this is a ridiculous number. If you want to try this with an Excel spreadsheet, use the formula =FV(0.1,2040,0,1) .

The problem is that compound interest is an exponential function, and increasing exponentials are bad. Think nuclear blast bad. There are many fables that warn about this, usually in the form of receiving one grain of rice on day 1, two grains on day two, 4 grains on day three, etc. Not long after, it quickly grows to be larger than the entire kingdom's food supply. The only difference here is that the interest rate is 100% and the compounding period is a day, so the ruse is discovered quickly. In the case of commercial interest, it takes a few generations, but the result is the same.

Compound Interest is at the heart of all modern investments including pensions. The savings you put away during your working life cannot possibly support you from age 65 to perhaps 90, but not to worry because they will grow at 7%. We must recognize that this is impossible.

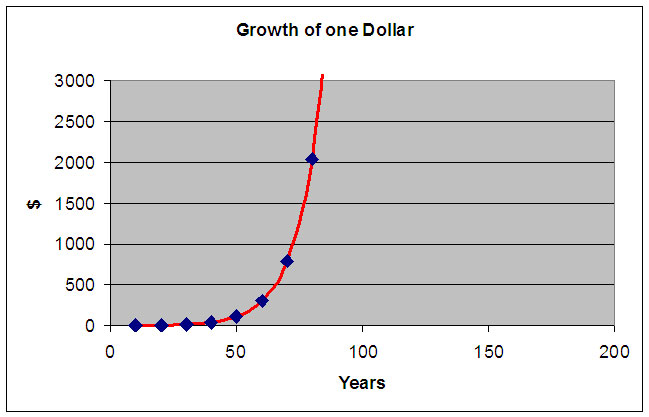
Notice the general shape of the exponential curve. It begins gently enough but then shoots skyward like a rocket. If this was an engineering problem, it would be considered "unstable" and the system would be redesigned to eliminate it.

Compound interest cannot be part of a sustainable society.

That brings us to another point -- why does the system seem ok and why did it work for our parents? There are two important points here. The first is that we have also had an exponential population growth so while the money supply has been growing, so has the population. That tends to maintain a sense of "proportion" and why we talk of bailouts in the trillions today as if that were normal -- a number unimaginable a generation ago.

 

The second point is that in the beginning of exponential growth, the numbers are small. Over your life time, your $1 could grow to $2000, and these are countable numbers. There doesn't seem to be anything outrageous. However, when you look at people that are further up the curve, old money, families and institutions that were millionaires in the great depression, their wealth has skyrocketed to astronomical levels. This has resulted in a core group of tightly interconnected powerful corporations that dominate worldwide commerce, and most of them are financial corporations. For a longer discussion of this important problem, see global corporate control.

Implicatations for Newfoundland Fiscal Policy

Debts with interest grow exponentially, so a province that gets into long term debt that can't be paid down rapidly ends up on the rocket section of the exponential curve where they can't even make interest payments. At that point, the province enters into economic slavery. Perhaps the debt seemed resonable based on projections of oil revenues, but one oil spill and all that could change.

This is no trivial matter. There is a global debt crisis right now (i.e. 2012) and will continue. See: sovereign-debt crisis, zerohedge, Iceland financial crisis, or search on Twitter for terms like default, revolt, revolution and you will find many excellent, but disturbing news articles.

Many debts were agreed to long ago by long dead politicians and have nothing to do with the current generation. When these debts become unpayable, you get a situation like current day Greece where a country can literally get taken over by financial institutions like the international monetary fund and central banks. Imagine having a premier appointed by the Bank of Canada to oversee the sale of provincial assets like Nalcor to pay down the debt so that the banking interests can get their money back plus interest.

The solution for the hopeless indebted is of course is to default. However, default opens up assets, like hydroelectric power plants, to confiscation or privatization. Any province that wants to avoid the problems we see now in Spain, Greece and much of the Europe needs to stay out of debt or be willing to default and engage in an epic battle to fight off legal and political vultures.

Solutions

The ultra rich are powerful institutions and people who have no intention of giving up their wealth so there are two solutions. One is total financial collapse and to start over with a new currency, perhaps silver coins that are traded and have no face value, just a weight. There is also an elegant mathematical solution to compound interest. Remember logarithms from high school?

The opposite of the exponential function is the logarithm. These two functions undo each other. For example log($100) is $2, log($million) = $6 and log($billion) is $9. That would make the Irving family 10 times richer than an ordinary person with $100 to her name. If you think this is brutal, remember that this is no more brutal than allowing the opposite, letting wealthy families to accumulate trillions over a few generations via compound interest and using that wealth to control the world.

In a game of monopoly, when someone gets all the money, they winner doesn't refuse to quit and insist the other players be slaves. It is simply game over.